Video from my YouTube channel

Subscribe to My You tube channel

Changes in length of axially loaded members

By -
Length of axially loaded members will change when they are subjected to axial load, members length will decrease when it is subjected to compression load, The length will increase for tension loads. to illustrate the concept we will begin with spring.
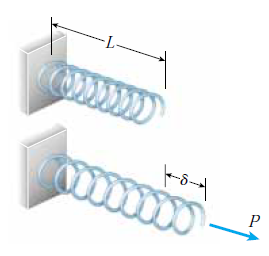
Let consider spring in figure 1, assuming that this spring is subjected to tension force equal P, the length of spring before elongation equal to L. after applying the force the length will increase by Î´, the total length after elongation will be L+δ. if the material is linearly elastic the load and elongation will be proportional
P=k*δ, Î´=f*P

The constant k is the stiffness of the spring and can be defined as the force required to make a unit of elongation. the constant f is the flexibility of the spring and can be defined as the elongation produced by a load of unit value.

k=P/δ, f=δ/P
k=1/f, f=1/k

flexibility can be determined easily by measuring the elongation for a known force, from flexibility stiffness of the spring can be calculated using the previous equation. 







Figure 1



Prismatic bar, a prismatic bar will elongate under tension and shorten under compression similarly as in springs, if the prismatic bar cross-section is constant through the length with area A, prismatic bar could have different cross-section as shown in figure 2, if the load act at the center of bar, then stress will be Ïƒ=P/A, the strain Îµ=δ/L, assuming the material linearly elastic and using hook law Ïƒ=E*ε, where E is the modulus of elasticity, elongation can be calculated using the following equation after rearranging hook's law

δ=P*L/(A*E)

The elongation is directly proportional to P and L, and inversely proportional to A and E, the product of A*E is known as axial rigidity. 
Figure 2
The stiffness and elongation for bar defined similarly as in spring, stiffness is the force required to produce a unit of elongation K=(AE)/L, flexibility know as the elongation generated from applying a load of unit value f=L/(AE)

Cables, cables are similar to bar and spring when they are subjected to tensile force, cables cannot resist compressive force and they have a little resistance to bending, cables are made from strands wound around a central strand and each strand made from many wires wound helically. the cross-section area of a cable is the total cross-section area of individual wires and it is known as effective-cross section area, the effective cross-section area is less than the area of a circle with the same diameter due to space between wires of a cable.
for a cable with the effective area and material similar to a bar, the elongation of cable will be more, because the wire will tighten up as in rope fibers, the modulus of elasticity for a cable known as effective modulus of elasticity is less than the modulus of elasticity for material which is made, the effective modulus of elasticity for a steel cable is around 140GPa (20,000Psi) while the modulus of elasticity for steel is  around 210 GPa, 30,000Psi, the elongation for cable shall be calculated using effective cross-section area and effective modulus of elasticity.



Comments

Post a Comment

Videos from My Youtube channel

Popular posts from this blog

Pile cap

By -
Image
Pile cap used to transfer the loads from superstructure to the piling. The pile cap is thick concrete mat rests on piles. It is part of the foundation and used to distribute the loads over the piles. Piles used when the soil bearing is not enough to carry loads of the structure. Figure 1  construction management: concrete construction bridge construction:How to become a bridge engineer Pile cap construction procedure Excavation around piles: After 7 days of the casting of the pile the concrete will gain approximately, 70% of it is compressive strength. The soil around piles will be removed to the required levels and dimension that allows construction of the pile cap. A step shall be made if the excavation is more than 1.5 m to prevent soil collapse. Figure 2 Pile head cutting: Concrete will be removed up to cut off level. If concrete under the cut off level is unsound. It shall be removed, and the pile will be repaired...
Read more

Binding wire for steel reinforcement

By -
Image
  Binding wires are used to tie steel bars together. These wires are playing a significant role in maintaining the reinforcement stability and rigidity. Wires are used to tie the steel bars at intersections points. By tying the steel bars together, we ensure that the steel bars will not move from their locations during construction or during the time of concreting. In slabs, binding wires are used to tie longitudinal and transverse bars together. In columns, it is used to tie vertical bars with stirrups.   Figure 1 There are various types of binding wires such as  Black annealed baling wire, Stainless Steel Binding Wire, and PVC coated binding wires.  Black annealed baling wire used to tie black steel. The popular size for black wire ranges from 16 to 22 gauges. Stainless steel binding wires are used to bind stainless steel reinforcement. Stainless steel reinforcement is used in a harsh environment where the black steel gets rusted quickly. Therefore, stainless ...
Read more

Repairing of concrete bulging

By -
Image
Improper designing and installation of formwork for a concrete member can result in concrete Bulging. During the placing and compacting of concrete, forms may deflect largely in the weak areas. The deflection of forms will cause concrete to expand at this location, and this known as concrete Bulging. Concrete Bulging will spoil the appearance of a concrete member. Therefore we should always ensure that the forms are appropriately installed, and it is sufficiently supported to resist the concrete during placing and compacting. Bulging should be repaired in case we couldn't avoid it. The solution of concrete Bulging, offset, or surface irregularities are surface grinding. However, excessive surface grinding can weaken the concrete.  Figure 1 If the bulging or surface irregularities are small, grinding of concrete surface will be sufficient to remove surface irregularities. If the Bulging of concrete is significant, then the concrete should be chipped, and the surface...
Read more

Casing for piles

By -
Image
The casing is a thick steel tube. The casing used extensively in construction drilled piles. The casing used to guide the drilling rig to drill the pile hole in the correct location. Also, it can be used to stabilize and prevent the soil from collapse until the concreting of the pile is completed. Furthermore, the casing will be used to hang the steel cage until the concrete is poured. The used casing should be thick and strong enough to resist the imposed stresses during the installation and removal process for temporarily casing. The casing should be clean, smooth, free from encrusted concrete, or any damage that can affect the size and shape of the pile. Figure 1 The casing length will vary according to the purpose of use and the type of the soil. If supporting fluids are used to stabilize the soil, the casing will be used as a guide for the drilling rig. Therefore, the casing length will be short. If the casing is used to support the soil, the casing length should be s...
Read more

Bridge Diaphragm

By -
Image
The main function of diaphragms is to provide a stiffening effect to deck slab in case bridge webs are not situated directly on top of bearings. Therefore, diaphragms may not be necessary in case bridge bearings are placed directly under the webs because loads in bridge decks can be directly transferred to the bearings.On the other hand, diaphragms also help to improve the load-sharing characteristics of bridges. Diaphragms also contribute to the provision of torsional restraint to the bridge deck. Diaphragm will be used also to resist stresses that occurs during the post-tensioning process. Post-tensioning hardware are casted inside the diaphragm. Figure 1 construction management: concrete construction bridge construction:How to become a bridge engineer Types of diaphragm: Diaphragm at the span end: this type of diaphragms will be located between two bridge spans or at the bridge abutments. Expansion joints will be used to connect bridge spans. This ...
Read more

Driven piles

By -
Image
Driven piles have been used widely as a foundation for different structures. In this type of foundation. Piles are driven to soil mechanically using a hammer. There are enormous types of driven piles. construction management: concrete construction bridge construction:How to become a bridge engineer  Steel H Piles Section of H-pile consists of flange and web, as shown in figure no:1. They are driven using a high impact hammer, and the likelihood of pile damage is low. H-piles resists the loads through skin friction or end bearing or both. Since the displacement of soil is minimal, this type of piles can be driven into dense granular soil or stiff clay layers. Although some problem during driving may arise. Plugging is one of them. After driving the pile to a certain level. soils will adhere to pile web and between the flange creating a closed end. This will change the pile to displaced pile and complicate pile driving. H-piles should be protect...
Read more

Lateral support and skin reinforcement for reinforced beam

By -
Image
The buckling of the unsupported beam with any dimension even narrow and deep is a rare occurrence. If the beam subjected to appreciable lateral torsion. ACI code [10.4.1] stating that lateral support is not required to placed closer than 50 times b, where b is the least width of compression face or flange.however, it must be considered in determining the maximum spacing for lateral support. construction management: concrete construction bridge construction:How to become a bridge engineer Skin Reinforcement for Deep Beams ACI code [10.6.7] stating that skin reinforcement shall be added for beams with depth h is 400 mm or more, the purpose of this reinforcement is to control cracks in the upper part of the tension zone, this reinforcement will be distributed in both vertical faces of the beam within a distance of h/2 from the tension zone as shown in figure 1, Figure 1 construction management: concrete construction bridge construction:How to become a br...
Read more

Non-Working Pile Testing and Static Compression Load Test

By -
Image
Purpose of Non-Working Piles Non-working piles are constructed at the beginning of the project to evaluate soil bearing capacity and determine the maximum settlement. These piles are loaded up to twice the working load. Based on the test results, the pile length may be adjusted. If the measured settlement exceeds the allowable limit, the pile length will be increased accordingly. construction management: concrete construction bridge construction:How to become a bridge engineer Static Compression Load Test The static compression load test provides insight into how the pile responds to loading. Settlement is measured at different load increments to determine the appropriate pile design length. The maximum applied load is typically twice the working load (2 × working load) or as specified by local standards. The following steps outline the testing procedure: A. Steel Cage Preparation The steel reinforcement cage is prepared in accordance with the approved shop drawings. ...
Read more

Equivalent Single Axle Load (ESAL) in Pavement Design

By -
Image
In pavement engineering, determining the Equivalent Single Axle Load (ESAL) is a crucial step in designing a durable and efficient roadway. ESAL simplifies the complexity of mixed traffic loading by converting it into a single representative value, allowing engineers to assess the cumulative impact of various vehicle types on pavement performance. The Concept of ESAL Roadways are subjected to traffic from a wide range of vehicles, each with different axle configurations and weights. Since these vehicles exert varying degrees of stress on the pavement, ESAL provides a standardized measure to represent their combined effect. The core idea behind ESAL is to express the cumulative traffic load on a road pavement as an equivalent number of passes of a standard axle load. This standard axle load is typically set at 80 kN . For example, if a road is subjected to an ESAL value of 10,000,000 , it means the pavement experiences the same level of stress as 10 million passes of a single standard...
Read more

Chamfers for concrete structures

By -
Image
In general, the edges of concrete members are chamfered. Usually during the process of installing forms for a structural member such as pile cap, a chamfer a PVC chamfer as shown in figure no:1 installed at the edges of the structural members. These chamfers will form smooth slanted edges, as shown in figure no:2. The size of chamfers can be 20x20mm or more as shown on the contract drawing. The PVC chamfer fixed to forms by nails. We should ensure that the PVC chamfer fixed in their correct locations, and we should ensure that the PVC chamfer is straight and not moving up or down. Checking the alignment and location of PVC chamfer is important, so the chamfered concrete edge will have a good appearance after the form’s removal.  Figure 1 Figure 2 Chamfering the concrete edges done for many reasons such as aesthetics, some suggesting that the chamfering of concrete edges done for safety to reduce the damage of objects due to impact with sharp concrete edges. Actually, ...
Read more