Changes in lengths for axially loaded members under nonuniform conditions
For a prismatic bar loaded at both ends only, elongation or shortening can be calculated using this equation 𝛿=(P*L)/(AE). in some cases the prismatic bar will not be loaded only at endpoints, the load will be applied at an intermediate point as shown in figure 1. for such case we can calculate the change in length by algebraically summing the elongation or shortening of each segment
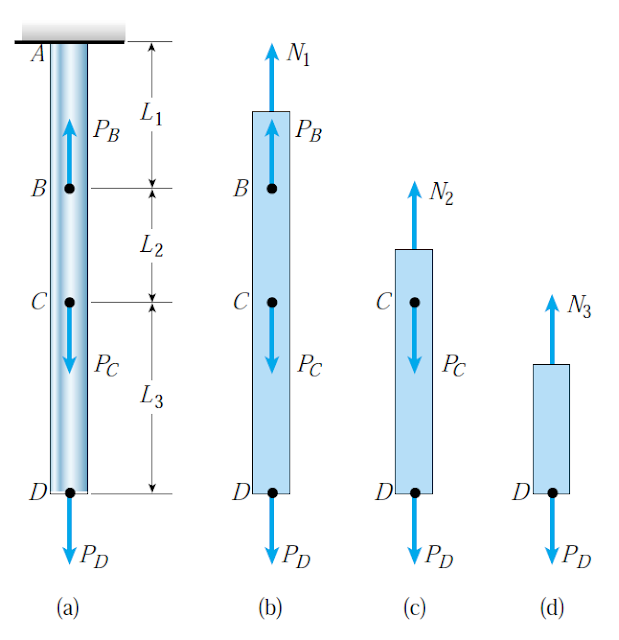
For a prisimatic bar in figure 1-a, the procedure will be as follows:
Figure 1
For a prisimatic bar in figure 1-a, the procedure will be as follows:
- determine the separate segments for the bar, segments AB, BC, CD.
- Determine the internal axial force at each segment N1, N2, N3 from the free body diagram.
- For first segment N1 from free body diagram (figure 1-b) N1=PD+PC-PB
- for second segment N2=PD+PC (figure 1-c)
- For third segment N3=PD (figure 1-d)
- then determine the change of length for each segment 𝛿1=(N1*L1)/(A*E), 𝛿2=(N2*L2)/(A*E), 𝛿3=(N3*L3)/(A*E)
- add 𝛿1, 𝛿2 and 𝛿3 to obtain the total change in length for the bar
Σ𝛿=𝛿1+𝛿2+𝛿3
a similar approach will be followed for a bar consisting of prismatic segments, the internal axial force for each segment should be determined, also the change of length for each segment should be determined 𝛿1=(N1*L1)/(A*E) but the used area should be for the segment under investigation, also the modulus of elasticity(E) for the segment under investigation should be used if different materials used.
Figure 2

















Comments
Post a Comment