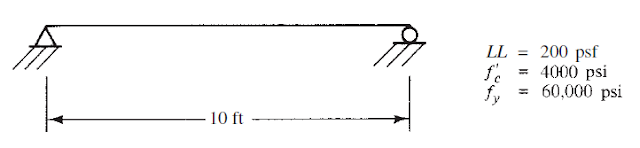
Example 1: Design of one-way slab

Design a one-way slab for the inside of a building using the span, loads, and other data given in Figure 1. Normal-weight aggregate concrete is specified with a density of 145 pcf. (assuming cover 3/4 in)

Figure 1

The minimum thickness for one-way slab simply supported=L/20 using table 1(ACI 9.5.2.1)

h=10/20=0.5=6in

Table 1

construction management: concrete construction

bridge construction:How to become a bridge engineer

now we will calculate d=(6-3/4(cover)-1/4(estimated as half diameter of reinforcement)

d=5 in 

now we will calculate dead load

concrete density=145pcf, Usually 5 pcf is added to account for the weight of reinforcement, so 150

pcf is used in calculating the weight of a normal-weight concrete member.

dd=(6in*ft/12)*1*150/=75 lb/ft

LL=200*(1)=200lb/ft

Wu=1.2*75+1.6*200=410 lb/ft

maximum moment for simply supported span =(Wu*L^2)/8

=(410*10^2)/8=8/=5,125lb-ft=61,500lb-in

now we can calculate ρ

  ρ=(0.85*fc'/fy)*(1-√(1-(2*Rn/0.85*fc'))

Rn=Mu/(ϕ*b*d^2)

Rn=(61500)/(0.9*12*(5^2))=227.7

  ρ=(0.85*4000'/6000)*(1-√(1-(2*227.7/0.85*4000))=0.00393

checking for ρmin
ρmin=(3√fc'/fy) and not less than 200/fy
ρmin=3*√4000/6000 and not less than 200/60000
ρmin=0.0003
ρ>ρmin   ok

As=0.00393*b*d
As=0.00393*12*6=0.282 in2/ft
Use #4 @ 10 in. from Table A.6 (As = 0.24 in2/ft)
Spacing < maximum of 18 in. as per ACI 7.6.5



checking for ϕ=0.9??

(εs+0.003)/d=0.003/C
C=a/β1
β1=0.85 for fc'=4000 psi
a=(fy*As)/(0.85*fc'*b)
a=0.35
c=0.35/.85=0.415

εs=0.033>0.005 so the section is tension control..............ok


Transverse direction-shrinkage and creep

steel G60
then As=0.0018*b*d=0.0018*12*6=0.1296in2

Use #3 @ 10 in. (0.13 in2/ft)

Use #3 @ 10 in. (0.13 in2/ft)  ok

The bar #4 is placed below bar #3 because the effective depth is important for main reinforcement calculation (flexural calculation)